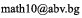Cubic and Quartic Equations

Cubic Equation x³ + a₁x² + a₂x + a₃ = 0

Let $Q = \frac{3a_2 - a_1^2}{9}$

$R = \frac{9a_1a_2 - 27a_3 - 2a_1^3}{54}$

$S = \sqrt[3]{R+\sqrt{Q^3+R^2}}$

$T = \sqrt[3]{R-\sqrt{Q^3+R^2}}$

Solutions:
$\begin{cases}x_1 = S + T - \frac{1}{3}a_1 \\ x_2 = -\frac{1}{2}(S+T) - \frac{1}{3}a_1+\frac{1}{2}i\sqrt{3}(S-T) \\ x_3 = -\frac{1}{2}(S + T) - \frac{1}{3}a_1-\frac{1}{2}i\sqrt{3}(S-T) \end{cases}$

If a₁, a₂, a₃ are real and if D = Q³ + R² is the discriminant, then
(i)     one root is real and two complex conjugate if D > 0
(ii)     all roots are real and at least two are equal if D = 0
(iii)     all roots are real and unequal if D < 0.
If D < 0, computation is simplyfied by use of trigonometry.

Solutions if D < 0 :
$\begin{cases} x_1 = 2 \sqrt{-Q} \cos \left( \frac{1}{3} \theta \right) \\ x_2 = 2 \sqrt{-Q} \cos \left( \frac{1}{3} \theta + 120^{\circ} \right) \\ x_3 = 2 \sqrt{-Q} \cos \left( \frac{1}{3} \theta + 240^{\circ} \right) \end{cases}$

where $\cos \theta = -\frac{R}{\sqrt{-Q^3}}$

            x₁ + x₂ + x₃ = -a₁,      x₁x₂ + x₂x₃ + x₃x₁ = a₂,      x₁x₂x₃ = - a₃
where x₁, x₂ , x₃ are the three roots.

Quartic equation x⁴ + a₁x³ + a₂x² + a₃x + a₄ = 0.

Let $y_1$ be a real root of the cubic equation
(1)   $y^3 - a_2y^2 + (a_1a_3 - 4a_4)y + (4a_2a_4 - a_3^2 - a_1^2a_4) = 0$

Solutions:    The roots of
$z^2+\frac{1}{2}(a_1 \pm \sqrt{a_1^2-4a_2+4y_1})z+\frac{1}{2}(y_1 \pm \sqrt{y_1^2 - 4a_4})=0$

If all roots of (1) are real, computation is simplified by using that particular real root which produces all real coefficients in the quadratic equation.

where x₁,x₂,x₃,x₄ are the four roots.