Basic Differential Equations and Solutions

Separation of variables
f₁(x)g₁(y)dx + f₂(x)g₂(y)dy = 0

Solution
$\int\frac{f_1(x)}{f_2(x)}dx + \int\frac{g_2(y)}{g_1(y)}dy = c$

Linear first order eqaution
dx/dy + P(x)y = Q(x)

Solution
$y e^{\int P dx} = \int Q e^{\int P dx} dx + c$

Bernoulli's equation
dy/dx + P(x)y = Q(x)yⁿ

Solution
$v e^{(1-n) \int P dx} = (1-n) \int Q e^{(1-n) \int P dx} dx + c$
where v = y^1-n.
If n = 1, the solution is
$ln y = \int (Q - P ) dx + c$

Exact equation
M(x,y)dx + N(x,y)dy = 0, where ∂M/∂y = ∂N/∂x.

Solution
$\int M \partial x + \int (N - \frac{\partial}{\partial y}\int M \partial x) dy = c$
where ∂x indicates that the integration is to be performed with respect to x keeping y constant.

Homogeneous equation
dy/dx = F(y/x).

Solution
$ln x = \int \frac{dv}{F(v) - v} + c$where v = y/x.If F(v) = v, the solution is y = cx.

yF(xy)dx + xG(xy)dy = 0

Solution
$ln x = \int \frac{G(v) dv}{v \{G(v) - F(v)\} } + c$where v = xy. If G(v) = F(v), the solution is xy = c.

Linear, homogeneous second order eqaution
d²y/dx² + a(dy/dx) + by = 0 , a,b are real constant.

Solution
Let m₁, m₂ are roots of m² + am + b = 0.Then there are three cases

Case 1.     m₁,m₂ real and distinct:
$y = c_1 e^{m_1 x} + c_2 e^{m_2 x}$

Case 2.     m₁,m₂ real and equal:
$y = c_1 e^{m_1 x} + c_2 x e^{m_1 x}$

Case 3.     m₁ = p + qi,m₂ = p - qi:
$y = e^{px} (c_1 \cos qx + c_2 \sin qx)$

Linear, nonhomogeneous second order equation
d²y/dx² + a(dy/dx) + by = R(x), a, b are real constants.

Solution
There are three cases corresponding to the above ones.

Case 1

Case 2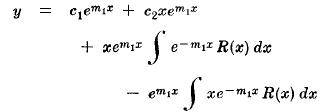

Case 3

Euler or Cauchy equation
x²d²y/dx² + a(dy/dx) + by = S(x) .

Solution
Putting x = e^t, the equation becomes
d²y/dt² + (a - 1)(dy/dt) + by = S(e^t)
and can then be solved as the above two entries.

Bessel's equation
x²d²y/dx² + x(dy/dx) + (λ²x² - n²)y = 0.

Solution
y = c₁J_n(λx) + c₂Y_n(x).

Transformed Bessel's equation
x²d²y/dx² + (2p + 1)x(dy/dx) + (α²x^2r + β²)y = 0.

Solution
$y = x^{-p} \{c_1 J_{q/r} (\frac{\alpha}{\gamma}x^r) + c_2 Y_{q/r} (\frac{\alpha}{\gamma}x^r)\}$
where q = √p² - β².

Legendre'e equation
(1 - x²)d²y/dx² - 2xdy/dx + n(n + 1)y = 0.

Solution
y = c₁P_n(x) + c₂Q_n(y).